
Dr. Michael Hoff
ehem. Hahn 
Fachrichtung Mathematik
Campus, Gebäude E2 4
Universität des Saarlandes
66123 Saarbrücken
Germany
Room: 428
Phone: +49681/302-3227
Email: hahn@math.uni-sb.de
Research Interests:
Preprints:
Papers:
Macaulay2-files:
| K3surfaces.m2 | Documentation | Explicit equations of K3 surfaces |
| RelativeCanonicalResolution.m2 | Documentation | Computation of relative canonical resolution and Eagon-Northcott type complexes |
| ExtensionsAndTorsWithLimitedDegree.m2 | Documentation | Computation of the homogeneous components of the graded modules Ext^i(M,N) and Tor^i(M,N) with a fixed degree limit |
| M2codeThesisHoff.m2 / constructionOfTheOsculatingCone.m2 | M2 code for my thesis | |
| relativeCanonicalResolutionsAndK3Surfaces.m2 | ancillary file to "Moduli of lattice polarized K3 surfaces via relative canonical resolutions" | |
| surface.m2, surface-char0.m2 | ancillary files to "New examples of rational Gushel-Mukai fourfolds" | |
| ancillary file to "Brill-Noether general K3 surfaces with the maximal number of elliptic pencils of minimal degree" | ||
| ConstructingEllipticK3Surfaces-supportingM2files.zip | ancillary files to "Unirational moduli spaces of some elliptic K3 surfaces" |
|
| CalabiYauThreefold.m2 | ancillary file to "On the numerical dimension of Calabi-Yau 3-folds of Picard number 2" |
Experiments concerning relative canonical resolutions:
The webpage (click here) lists all experimental results concerning the shape of relative canonical resolutions done with the Macaulay2-package "RelativeCanonicalResolution.m2"
Pictures and Animations:
| To a general tetragonal canonical curve C of genus 6, we can associate a trigonal curve as follows. There is a pencil of planes intersecting C in the given g^1_4. Four different points in a plane has six connection lines which intersect in three further points. These further intersection points sweep out a trigonal curve C'. The union of C and C' is the osculating cone to the Brill-Noether locus W^0_4(C) at the given point g^1_4 of W^1_4(C). We denote by S the surface consisting of the connection lines swept out by the pencil of planes. Here you can see a real picture of the surface S with a highlighted plane. | 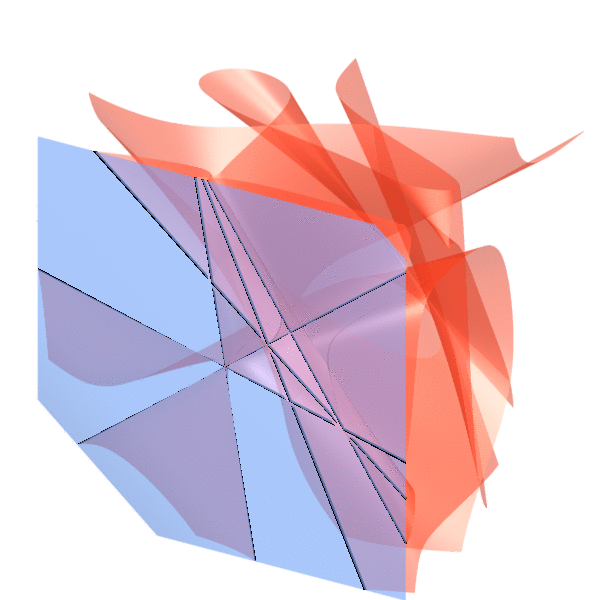 |
An animation of the surface S and a moving plane can be found here. The animation based on an example in "The osculating cone to special Brill-Noether loci".
Teaching:
Education: