Prof. Dr. Roland Speicher
Felix Leid
Free Probability / Freie Wahrscheinlichkeitstheorie
(Winter term 2018/2019)News
- The typed lecture notes for this course are available
- The Slides of lecture 21 (21.01.2019) are online .
- A Blog for the lecture is now online
Lecture
Monday, 10 -- 12, and Wednesday, 10 -- 12Room: Seminarraum 6 (SR6) in building E2.4
|
Free probability is a quite young mathematical theory with many avatars.
It started in the theory of operator algebras, showed its beautiful combinatorial
structure via non-crossing partitions, made contact with the world of random
matrices, and reached out to many other subjects like representation theory of large
groups, quantum groups, invariant subspace problem, large deviations, quantum
information theory, subfactors, or statistical inference. Even in physics and engineering,
people have heard of it and find it useful and exciting.
This course is in a sense a continuation of both the classes “Random Matrices” and “Operator Algebras” from the summer term 2018, as it deals with the concept of “freeness” or “free independence”, which appears both in the limit of random matrices as well as for important classes of von Neumann algebras. On the other hand, freeness is an important concept for its own sake which deserves to be investigated independently of its random matrix or operator algebraic roots. That’s what we will do in this course; we will, in particular, look at the combinatorial, analytical and probabilistic structure of freeness. 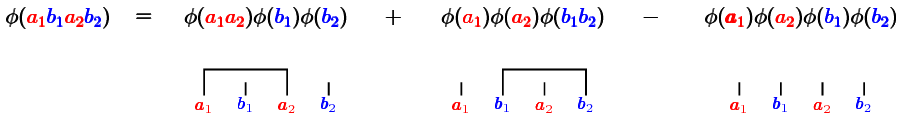
|
Lecture Announcement
Material
- The Lecture is recorded on video and the recordings are online available .
- Handwritten lecture notes can be found here .
- There is blog for this lecture, where you can make comments and ask questions related to the lecture.
Exercises
- Date & time : Monday 14 - 16
- Place : Seminar room 10 (SR10)
- Tutor : Alexander Mang (alex.mang[at]t-online.de)
- Mailbox: 114
Office hours
Felix Leid : By appointment Alexander Mang : Wednesday, 12:00 - 13:00, Room 317Problems
Exercise Sheet 1
Exercise Sheet 2
Exercise Sheet 3
Exercise Sheet 4
Exercise Sheet 5
Exercise Sheet 6
Exercise Sheet 7
Exercise Sheet 8
Exercise Sheet 9
Exercise Sheet 10
- D.-V. Voiculescu, N. Stammeier, M. Weber (eds.): Free Probability and Operator Algebras,
Münster Lectures in Mathematics, EMS, 2016 - James A. Mingo, Roland Speicher: Free Probability and Random Matrices.
Fields Institute Monographs, Vol. 35, Springer, New York, 2017. - A. Nica, R. Speicher: Lectures on the Combinatorics of Free Probability.
Cambridge University Press, 2006 - Fumio Hiai and Denis Petz: The Semicircle Law, Free Random Variables, and Entropy,
ISBN 0-8218-2081-8 - Voiculescu, D. V.; Dykema, K. J.; Nica, A.: Free random variables.
CRM Monograph Series, 1. American Mathematical Society, Providence, RI, 1992 - Terence Tao, 254A, Notes 5: Free probability, course notes for graduate course on "Topics in random matrix theory"
- Speicher, R.: Free Probability Theory; and its Avatars in Representation Theory, Random Matrices, and Operator Algebras;
also Featuring: Non-commutative Distributions, Jahresber. Dtsch. Math. Ver. (2017) 119: 3.
https://doi.org/10.1365/s13291-016-0150-5 - Novak, Jonathan. "Three lectures on free probability." Random matrix theory, interacting particle systems, and integrable systems 65 (2014): 309-383. https://arxiv.org/pdf/1205.2097.pdf
- Lecture Notes on Free Probability. Vladislav Kargin. https://arxiv.org/pdf/1305.2611.pdf
- Notes on Free probability Theory. Dimitri Shlyakhtenko https://arxiv.org/pdf/math/0504063.pdf
- Lecture notes Free Probability and Combinatorics. Michael Anshelevich.
updated: 31 Oct 2018 Felix Leid

